Chaos Theory: A Unpredictable World of Mathematics and Physics 

Introduction to Chaos Theory

    Chaos theory is a fascinating field of study that explores how systems, which might seem random and unpredictable, are actually governed by underlying patterns and rules. At its heart, chaos theory deals with deterministic systems—systems where future behavior is determined by their initial conditions, yet their outcomes are highly sensitive to small changes. This sensitivity is famously called the butterfly effect, where a tiny event, like a butterfly flapping its wings, could potentially cause a tornado in a distant place. 

In both mathematics and physics, chaos theory shows how even simple systems can evolve into something incredibly complex and unpredictable over time. This randomness, however, is not due to chance but is a result of the system's complex dynamics. 

Chaos Theory. 

Chaos Theory in Mathematics

Mathematically, chaos theory is rooted in non-linear equations, which are equations that do not form straight lines when graphed. Unlike linear systems, where small changes lead to proportional outcomes, non-linear systems can produce wildly different outcomes based on even the smallest differences in their starting points. A well-known example of a chaotic system is the logistic map, a mathematical formula used to describe population growth. The logistic map is expressed as:

$$x_{n+1} = r x_n (1 - x_n)$$

Here:

• $x_n$ is the population at time $n$,
• $r$ is a growth rate constant,
• $x_{n+1}$ is the population at the next time step.

This equation looks simple, but for certain values of $r$, the system becomes chaotic. Even a tiny change in the initial population can lead to drastically different future outcomes.

Chaos Theory in Physics

In physics, chaos theory appears in systems that are deterministic but unpredictable. One of the most famous chaotic systems is weather. Weather systems are governed by the laws of physics, yet we find it difficult to predict the weather accurately for more than a few days. This is because the system is highly sensitive to its initial conditions—a small difference in atmospheric conditions can lead to entirely different weather patterns.

Another example is the double pendulum. While a single pendulum swings back and forth in a predictable way, attaching a second pendulum to the first creates a system where the motion becomes unpredictable and chaotic, despite both pendulums being governed by Newton's laws of motion.

Hypotheses and Experiments in Chaos Theory

One of the key hypotheses in chaos theory is the idea that chaos is deterministic, not random. This means that, in theory, if we had perfect information about the initial conditions of a chaotic system, we could predict its future behavior. However, in practice, it is almost impossible to measure initial conditions with perfect accuracy, and even tiny inaccuracies grow over time, making long-term prediction impossible.

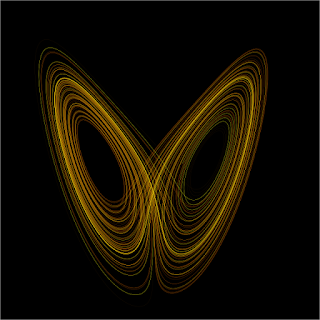
Edward Lorenz, a meteorologist, conducted one of the most famous experiments related to chaos theory in the 1960s. He was using a simple computer model to simulate weather patterns. One day, he tried to repeat a simulation but entered the initial conditions with slightly less precision. Instead of getting the same result, the weather pattern diverged dramatically, illustrating what we now call the Lorenz attractor and the butterfly effect. Lorenz's work showed that even systems governed by deterministic laws could behave unpredictably.

In terms of experiments, chaos theory can be seen in everyday life. The motion of fluids, the growth of populations, and the swings of financial markets all exhibit chaotic behavior. These systems follow mathematical rules, but predicting their behavior over long periods is impossible due to their extreme sensitivity to initial conditions.

Mathematical Expressions in Chaos Theory

Many systems in chaos theory are described using differential equations, which involve rates of change. One of the simplest examples is the Rossler attractor, a system of three linked equations that describe how a point moves through space in a chaotic way. The equations are:

$$\dot{x} = -y - z$$ $$\dot{y} = x + a y$$ $$\dot{z} = b + z(x - c)$$

Here, $a$, $b$, and $c$ are constants. Despite the simplicity of these equations, the behavior of the system is incredibly complex and chaotic for certain values of these constants.

Another famous set of chaotic equations is the Lorenz equations:

$$\frac{dx}{dt} = \sigma(y - x)$$ $$\frac{dy}{dt} = x(\rho - z) - y$$ $$\frac{dz}{dt} = xy - \beta z$$

These equations describe the flow of fluids (like air in the atmosphere) and produce chaotic behavior when certain conditions are met.

Fun Facts and Curious Insights

1. Fractals and Chaos: Chaotic systems often produce patterns called fractals. A fractal is a complex structure that looks the same at different scales. For example, the shape of a coastline is fractal-like: it appears jagged whether viewed from space or up close. Fractals are a visual representation of the infinite complexity of chaotic systems.

2. Chaos in Nature: Chaos theory isn’t limited to mathematics or physics. It is also present in biological systems. The rhythms of the heart, for example, can sometimes exhibit chaotic behavior, which can lead to arrhythmia.

3. The Butterfly Effect: The idea that small changes can lead to large, unpredictable consequences comes from chaos theory. In popular culture, this concept has been explored in movies like The Butterfly Effect and Jurassic Park, where chaos leads to unpredictable consequences.

4. Chaos in the Stock Market: Financial markets are another example of chaotic systems. They are influenced by countless factors, and small changes in one part of the market can lead to large and unpredictable swings in prices.

Hypotheses from Scientists

Several scientists have explored the implications of chaos theory. One hypothesis, proposed by Ilya Prigogine, is that chaos plays a role in the development of complex systems in nature, such as ecosystems and living organisms. He suggested that chaotic behavior might be necessary for the evolution of life, allowing systems to adapt to changing environments.

Another hypothesis involves the connection between chaos theory and quantum mechanics. Some researchers believe that the unpredictable behavior of subatomic particles could be described by chaotic processes, bridging the gap between classical and quantum physics.

Conclusion

Chaos theory reveals the hidden complexity in seemingly simple systems. By understanding chaos, scientists can better appreciate the unpredictable nature of the world around us, from weather patterns to stock markets and beyond. While chaos might seem like randomness, it is actually a rich and intricate system governed by precise mathematical rules. The beauty of chaos lies in its unpredictability and the way small changes can ripple across a system, producing complex and often surprising outcomes. 

References

• Edward Lorenz's work on the Lorenz attractor and the butterfly effect.
• Research on the logistic map and population dynamics.
• Studies of chaotic systems like the double pendulum and weather forecasting.
• Mathematical exploration of the Rossler and Lorenz attractors.
• Ilya Prigogine’s hypotheses on chaos and complex systems.

Chaos theory challenges us to think about the unpredictable side of nature, but it also opens up new ways of understanding the systems that influence our world. 

Finding the area of Sunspots: A Brief Abstract 

Abstract

Sunspots are a key feature in the study of solar activity, and their areas provide valuable insights into solar magnetic fields and their influence on space weather. The measurement of sunspot areas can be approached both observationally and mathematically. 

---

1. Introduction

Sunspots are dark regions on the Sun’s photosphere caused by intense magnetic activity that inhibits convection, making these regions cooler than their surroundings. These spots vary in size and number over time, reflecting changes in solar cycles, with implications for space weather and terrestrial climate. Calculating the area of sunspots is an essential part of solar observations, helping researchers understand the scale and impact of magnetic field disruptions on the Sun’s surface.

This article explores the methods to estimate sunspot areas using manual calculations, ranging from angular measurements to determining the fraction of the Sun's surface covered by sunspots.

---

2. Sunspot Size Representation

Sunspots are generally circular or elliptical, and their areas are often expressed in microhemispheres (µH), where one microhemisphere is equivalent to one-millionth of the Sun's visible hemisphere. Alternatively, sunspot areas can be described in terms of angular diameter, representing the angular size of the sunspot as seen from Earth.

The angular diameter is measured in radians, and this measurement can be converted into physical units to determine the actual area of the sunspot on the solar surface.

---

3. Theoretical Framework for Sunspot Area Calculation

3.1 Area of a Circle

Since sunspots approximate circular shapes, their area can be calculated using the standard formula for the area of a circle:

$$\text{A} = \pi \times \left(\frac{d}{2}\right)^2$$

Where:

• $A$ is the area of the sunspot.
• $d$ is the diameter of the sunspot.

This formula provides a direct method for determining the area if the physical diameter of the sunspot is known.

3.2 Converting Angular Size to Physical Size

Sunspot sizes are often given in angular diameter, and it is necessary to convert this angular measurement into the physical diameter of the sunspot. This conversion relies on basic geometry and the distance between the Earth and the Sun.

The angular size $\theta$ (in radians) is related to the actual diameter $d$ of the sunspot by the following equation:

$$d = \theta \times D$$

Where:

• $d$ is the physical diameter of the sunspot,
• $\theta$ is the angular diameter in radians,
• $D$ is the distance from the Earth to the Sun, approximately $1.496 \times 10^8$ km.

Once the physical diameter $d$ is determined, the area of the sunspot can be calculated using the formula for the area of a circle.

---

4. Sun’s Surface Area

To put the size of a sunspot into perspective, it is useful to compare it to the total surface area of the Sun. The surface area $A_{\text{Sun}}$ of a spherical object, such as the Sun, is given by:

$$A_{\text{Sun}} = 4\pi R^2$$

Where:

• $R$ is the radius of the Sun, approximately $6.96 \times 10^5$km.

Using this formula, the total surface area of the Sun can be calculated as approximately $6.09 \times 10^{12}$ square kilometers.

---

5. Fraction of the Sun’s Surface Covered by a Sunspot

The fraction of the Sun's surface area covered by a sunspot can be expressed as:

$$\text{Fraction} = \frac{\text{Area of Sunspot}}{\text{Surface Area of the Sun}}$$

This fraction provides a useful metric for understanding the relative size of the sunspot compared to the Sun's total visible surface. Even large sunspots tend to cover only a small fraction of the Sun’s surface.

---

6. Example Calculation

To illustrate the process, consider a sunspot with an angular diameter of 0.01 radians. The following steps outline how to calculate its physical size and compare it to the total surface area of the Sun.

Step 1: Calculate the physical diameter of the sunspot

Using the formula for converting angular size to physical size:

$$d = \theta \times D = 0.01 \times 1.496 \times 10^8 = 1.496 \times 10^6 \text{ km}$$

Step 2: Calculate the area of the sunspot

Using the formula for the area of a circle:

$$A_{\text{sunspot}} = \pi \times \left(\frac{1.496 \times 10^6}{2}\right)^2 = \pi \times (7.48 \times 10^5)^2 \approx 1.76 \times 10^{12} \text{ km}^2$$

Step 3: Compare to the Sun’s surface area

The total surface area of the Sun is approximately $6.09 \times 10^{12}$ km². The fraction of the Sun’s surface covered by this sunspot is:

$$\text{Fraction} = \frac{1.76 \times 10^{12}}{6.09 \times 10^{12}} \approx 0.289$$

This means that, in this example, the sunspot would cover roughly 28.9% of the Sun’s visible surface, although this is an unusually large sunspot for illustrative purposes.

---

7. Conclusion

Accurately calculating the area of sunspots is crucial for understanding solar dynamics and their broader implications on solar-terrestrial relations. The conversion of angular diameter to physical size and area provides a straightforward method for determining the extent of sunspot coverage. Additionally, comparing the sunspot area to the total surface area of the Sun offers insight into the scale of solar magnetic phenomena.

The mathematical approach presented here offers a foundation for manual calculations and can be further refined through more advanced observational techniques. 

---

References

• Hathaway, D. H. (2015). The Solar Cycle. Living Reviews in Solar Physics, 12(1), 4.
• Schrijver, C. J., & Zwaan, C. (2000). Solar and Stellar Magnetic Activity. Cambridge University Press.
• Petrovay, K. (2010). Solar Cycle Prediction. Living Reviews in Solar Physics, 7(6). 

Who was Julius Caesar?

A cunning military commander who ascended through the ranks of the Roman Republic, Gaius Julius Caesar eventually proclaimed himself dictator for life, upending the very foundations of Rome. Through bold political changes, Julius Caesar turned Rome from a republic into an empire. 

Julius Ceaser De Rome. 

Early Life and Family Background

• Julius Caesar was born on July 12 or 13, 100 B.C.E. into a noble Roman family, the gens Julia, which claimed descent from the goddess Venus. Despite his noble heritage, his family was not particularly wealthy or politically influential at the time. 
• Caesar grew up during a period of political instability in Rome, where the Roman Republic was in chaos, marked by social unrest, civil wars, and political corruption. This environment would later shape his political ambitions and strategies. 

Rise to Power: Political and Military Beginnings

• Caesar's early political career began with a series of modest posts in the Roman government. His military ambitions took him to Spain, where he served as governor. His time in Spain marked the beginning of his rise as a prominent figure in Roman politics.
• Upon returning to Rome, Caesar formed strategic alliances with powerful leaders. In 60 B.C.E., he joined forces with Pompey the Great, a celebrated general, and Marcus Licinius Crassus, the wealthiest man in Rome, creating the First Triumvirate. This political alliance allowed him to rise rapidly within Roman politics.

Conquest of Gaul (58–51 B.C.E.)

• As governor of Gaul (modern-day France and Belgium), Caesar led Roman troops in a series of military campaigns that expanded Roman control over the region. Over the course of eight years, he conquered various Gallic tribes, exploiting their internal divisions to secure victory.
• His most significant triumph came with the defeat of Vercingetorix, a powerful Gallic leader. After his defeat, Vercingetorix was paraded through Rome in chains and later executed. Caesar's campaign in Gaul significantly boosted his military power, wealth, and popularity in Rome, making him one of the most powerful figures in the Republic.

Crossing the Rubicon and Civil War (49 B.C.E.)

• In 49 B.C.E., Caesar defied the Roman Senate’s order to disband his army and return to Rome as a private citizen. Instead, he led his army across the Rubicon River, a symbolic act of rebellion against the Senate. This marked the beginning of a civil war between Caesar and his rival, Pompey, who had aligned with the Senate.
• Caesar’s forces quickly defeated Pompey’s army in Italy. Pompey fled to Greece, where Caesar pursued him. Despite Pompey’s army being larger, Caesar defeated him at the Battle of Pharsalus in 48 B.C.E. Pompey then fled to Egypt, where he was assassinated upon his arrival.

Caesar and Cleopatra

• After Pompey’s death, Caesar became entangled in the Egyptian political conflict. He formed a political and romantic alliance with Cleopatra, the Queen of Egypt. Together, they had a son named Caesarion.
• Caesar’s support of Cleopatra helped her solidify her rule in Egypt. He also spent some time in Egypt consolidating his power before returning to Rome.

Caesar’s Dictatorship

• Upon returning to Rome in 46 B.C.E., Caesar declared himself dictator, a role that gave him almost unlimited power. His reforms during this period included:
  • Enlarging the Senate to include representatives from across the empire.
  • Reducing Rome’s debts, providing relief to many Roman citizens.
  • Granting citizenship to foreigners, expanding Roman influence and inclusivity.
  • Public works projects, including the construction of the Forum Ilium and the rebuilding of Carthage and Corinth.
• These reforms, though beneficial to many, caused concern among Roman elites, especially the Senate. They feared Caesar was amassing too much power and might declare himself king.

Assassination of Caesar

• In February 44 B.C.E., Caesar declared himself dictator for life, a move that outraged many senators who believed in the Roman Republic’s tradition of limited power for its leaders. This declaration, along with Caesar’s increasing authority and ambitions, led to a conspiracy to end his life.
• On March 15, 44 B.C.E., the Ides of March, Caesar was assassinated by a group of senators, including Marcus Junius Brutus and Gaius Cassius Longinus. He was stabbed 23 times during a Senate meeting, signaling the end of his rule.
• Despite their efforts, the conspirators failed to restore the Republic. Caesar’s assassination plunged Rome into further chaos and civil war.

Caesar's Legacy and the Rise of the Roman Empire

• Caesar’s assassination did not end his influence over Rome. His grand-nephew and adopted heir, Octavian (later Augustus Caesar), ultimately emerged victorious in the subsequent power struggles. Octavian would become Rome’s first emperor, marking the transition from the Roman Republic to the Roman Empire.
• Posthumously, Caesar was deified by the Roman Senate and given the title Divine Julius, the first Roman to receive such an honor. His name, "Caesar," became synonymous with rulers in Rome and beyond, giving rise to the titles "Kaiser" in Germany and "Tsar" in Russia.

Impact on Roman History

• Julius Caesar’s life and actions transformed the Roman political landscape. His reforms and military conquests expanded Roman territory and centralized power within the state.
• His crossing of the Rubicon, a bold and irreversible decision, symbolized the end of the Roman Republic and set the stage for the rise of the Roman Empire.
• Caesar’s ambitious pursuit of power, combined with his political reforms and military victories, left an enduring legacy that shaped the course of Roman and world history.  

Schrödinger’s Cat Experiment: A Quantum Mystery.

Schrödinger’s Cat Experiment: A Quantum Mystery. 

The Schrödinger's Cat experiment is one of the most famous thought experiments in the field of quantum mechanics, proposed by Austrian physicist Erwin Schrödinger in 1935. The experiment was designed to illustrate the peculiarities of quantum superposition and the paradoxes that arise when quantum systems are scaled to the macroscopic world. At this thought experiment is a curious question: how can something be both alive and dead at the same time? 

Schrödinger’s Cat Experiment: A Quantum Mystery. 

The Thought Experiment: A Cat in a Box

In Schrödinger’s original thought experiment, imagine a cat is placed inside a sealed box, which contains the following elements:

1. A radioactive atom (a quantum system that has a 50% chance of decaying in a given time).
2. A Geiger counter to detect radiation.
3. A vial of poison.
4. A hammer connected to the Geiger counter that, if radiation is detected, will release the hammer and break the vial, killing the cat.

If the atom decays, the Geiger counter detects it, causing the hammer to break the poison vial and kill the cat. If the atom does not decay, the cat remains alive. The atom's decay is a quantum event, meaning it can exist in a superposition of decayed and undecayed states. But here’s where things get strange: according to quantum mechanics, until someone observes the system, the atom is in both states at once. As a result, the cat is theoretically both alive and dead at the same time.

This scenario creates a paradox when we think about how the quantum world (the behavior of the atom) and the macroscopic world (the fate of the cat) interact.

The Quantum Superposition

In quantum mechanics, particles like atoms exist in a superposition of all possible states until they are observed or measured. This idea is captured in Schrödinger's wave equation, which provides the mathematical description of the probability of finding a particle in a certain state.

Schrödinger's Equation (Time-Dependent):

$$i\hbar \frac{\partial}{\partial t} \Psi(x,t) = \hat{H} \Psi(x,t)$$

• $$Ψ(x,t): The wave function, which contains all possible information about the system.
• $\hat{H}$: The Hamiltonian operator, representing the total energy of the system.
• $\mathrm{<span\; style="color:\; \#274e13;\; font-family:\; arial;">\hslash :\; Reduced\; Planck’s\; constant.</span>}$
• $i$: The imaginary unit.

The wave function $\Psi(x,t)$ describes the quantum state of a system. Before measurement, the atom (and by extension, the cat) exists in a superposition of both decayed and undecayed states. The wave function collapses into a definite state (alive or dead) only when observed.

Cat Experiment. 

Copenhagen Interpretation: Observation and Collapse

One of the most widely accepted interpretations of quantum mechanics is the Copenhagen interpretation. According to this view, a system exists in superposition until it is observed. The act of measurement causes the wave function to "collapse" into one of the possible states. In the case of Schrödinger’s cat:

• Before opening the box, the cat is both alive and dead (superposition).
• Upon observation (when the box is opened), the wave function collapses, and the cat is either alive or dead.

This collapse represents the transition from the quantum world (where probabilities rule) to the classical world (where we experience definite outcomes).

The Many-Worlds Hypothesis

Another interpretation that seeks to resolve the Schrödinger's cat paradox is the Many-Worlds Interpretation. This theory suggests that every possible outcome of a quantum event actually happens, but in different parallel universes. In the case of the cat:

• In one universe, the cat is alive.
• In another universe, the cat is dead.

This interpretation eliminates the need for wave function collapse, as each possibility simply plays out in a separate universe.

Quantum Mechanics and the Measurement Problem

The Schrödinger’s Cat experiment highlights a central issue in quantum mechanics: the measurement problem. This problem deals with how and why observations cause a quantum system to collapse into a single state, transitioning from the probabilistic quantum world to the definite classical world.

Mathematical Expression for Superposition

To explain mathematically, the state of the cat can be represented as a superposition:

$$|\text{Cat}\rangle = \frac{1}{\sqrt{2}} \left( |\text{Alive}\rangle + |\text{Dead}\rangle \right)$$

Here, the cat is in a 50-50 superposition of being alive and dead. The probability of finding the cat in either state upon observation is 50%, but until the observation, the cat's true state is indeterminate.

Mathematical Representation

In quantum mechanics, the state of a system is described by a wave function, denoted by Ψ (psi). The wave function encodes all possible states of a system. In the case of the cat experiment, we can express the superposition of states mathematically as follows:

$$\Psi = \frac{1}{\sqrt{2}} \left( | \text{Alive} \rangle + | \text{Dead} \rangle \right)$$

Here, the cat is represented by the states $\mathrm{\mid }\text{Alive}⟩\; and\; \mid$$| \text{Dead} \rangle$, and the factor $\frac{1}{\sqrt{2}}$ ensures that the probabilities of both states sum to 1.

When the box is opened (the measurement is made), the wave function collapses into one of the two possible outcomes. The mathematical expression for the collapse is:

$$$$Ψcollapsed=∣Alive⟩orΨcollapsed=∣Dead⟩\Psi_{\text{collapsed}} = | \text{Alive} \rangle \quad \text{or} \quad \Psi_{\text{collapsed}} = | \text{Dead} \rangle

The probabilities are governed by the Born Rule, which states that the probability of an outcome is the square of the amplitude of the wave function for that state. For instance, if the cat has an equal chance of being alive or dead, the probabilities would be:

$$P(\text{Alive}) = |\langle \text{Alive} | \Psi \rangle|^2 = \frac{1}{2}, \quad P(\text{Dead}) = |\langle \text{Dead} | \Psi \rangle|^2 = \frac{1}{2}$$

This expresses that until the box is opened, both outcomes are equally likely.

The Role of Entanglement

Schrödinger’s Cat also illustrates the concept of quantum entanglement. The atom and the cat become entangled in such a way that the state of the cat is directly tied to the state of the atom:

• If the atom decays, the cat dies.
• If the atom does not decay, the cat remains alive.

Entanglement means the two systems (the atom and the cat) cannot be described independently of one another.

Fun and Curious Facts about Schrödinger’s Cat

1. Schrödinger’s Intention: Schrödinger originally devised this thought experiment to critique the Copenhagen interpretation, not to support it. He found the notion of a cat being both alive and dead absurd, using the thought experiment as a way to highlight the problems of applying quantum mechanics to everyday objects.

2. Applications to Quantum Computing: Schrödinger's cat has found a real-world application in quantum computing. The idea of superposition (being in multiple states at once) is at the heart of how quantum computers work, enabling them to perform complex calculations at unprecedented speeds.

3. Real-World Schrödinger’s Cats?: In recent years, scientists have been able to create real-world systems that mimic Schrödinger’s cat on a microscopic scale. They’ve used photons and other particles to show that quantum systems can indeed exist in superposition, though the "cat" in these experiments is far smaller and less complicated than a real animal.

4. Quantum Biology: Some scientists speculate that Schrödinger’s Cat may have applications in understanding quantum effects in biology, such as how plants use quantum mechanics in photosynthesis, where particles like electrons can exist in multiple places simultaneously.

Hypotheses and Interpretations Among Scientists

• Objective Collapse Theories: Some researchers propose that quantum systems naturally collapse into definite states after a certain amount of time or interaction with their environment. This avoids the need for observation to trigger the collapse.

• Quantum Darwinism: This hypothesis suggests that the classical world emerges through a process similar to natural selection, where certain quantum states are “selected” by their interactions with the environment, allowing them to become the definite states we observe.

Conclusion

Schrödinger’s Cat remains a profound symbol of the bizarre world of quantum mechanics. It reveals the strange and counterintuitive nature of the quantum realm, where particles can exist in multiple states, and observations change the nature of reality itself. While the cat is a thought experiment, its implications resonate throughout modern physics, from quantum computing to potential applications in quantum biology.

By exploring Schrödinger’s cat, we dive deeper into the mysteries of quantum superposition, measurement, and the transition from the microscopic quantum world to the macroscopic world we experience daily. The more we study, the more curious—and complex—this quantum world becomes. 

References:

1. Griffiths, D.J. (2004). Introduction to Quantum Mechanics. Pearson Prentice Hall.
2. Nielsen, M. A., & Chuang, I. L. (2010). Quantum Computation and Quantum Information. Cambridge University Press.
3. Everett, H. (1957). "Relative State Formulation of Quantum Mechanics." Reviews of Modern Physics.

These references will guide readers to explore the depth of quantum mechanics and its theoretical interpretations. 

"One can even set up quite ridiculous cases. A cat is penned up in a steel chamber, along with the following diabolical device... one would, according to the Copenhagen interpretation, have to admit that the cat is both dead and alive at the same time."
— Erwin Schrödinger.